若何倒饮料速率最快?从生活履历可以创造,倾斜瓶子能更快地倒出饮料,但是如果将瓶子全体倒过来,瓶口会产生气泡,并且饮料可能产生喷溅。对这个问题的研究或将对饮料行业和化工厂带来启示。
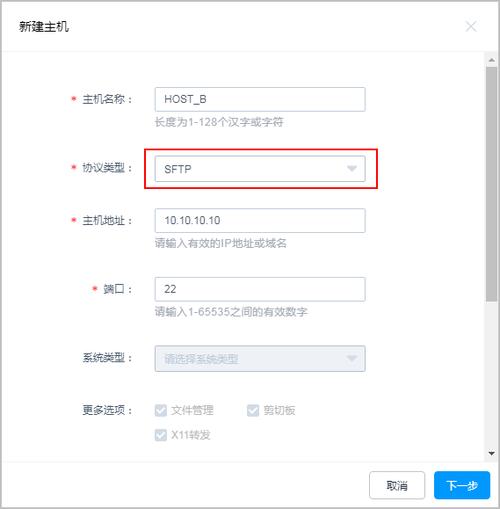
图为气泡在不同流体中的韶光蜕变,把稳每次吹完泡泡后排出的形状。图片来源:Lokesh Rohilla

来源 AMERICAN INSTITUTE OF PHYSICS
翻译 吴娜
审校 戚译引
你不雅观察过倒饮料的时候瓶子是若何排空的吗?印度理工学院(Indian Institute of Technology Roorkee)的研究职员探究了如何让瓶子更快地倒空,这对饮料业以外的许多领域都有广泛的影响。
几个世纪以来,人们对气泡进行了广泛的研究,包括列奥纳多·达·芬奇(Leonardo da Vinci)早期的研究成果。达·芬奇有一个著名的创造,即气泡呈正弦状上升。瓶口气泡产生的的动力学取决于液体的热物理性子、瓶体几何形状及其倾角。这些参数无可避免地交织在一起,使得空瓶动力学(bottle-emptying dynamics)成为气泡物理学家的下一个前沿研究方向。
在近期的 AIP 旗下期刊《流体物理学》(Physics of Fluids)中,Lokesh Rohilla 和 Arup Kumar Das 通过高速拍照剖析商业饮料瓶中的气泡动力学,探索了这种征象。图像剖析使他们能够将各种参数观点化,如液膜厚度,气泡长宽比,上升速率和瓶子排空模式。
Rohilla 说:“瓶子里的气泡动力学太繁芜了,很难研究,以是我们把气泡界面成长分为不同的阶段来理解。”
众所周知,如果你增大瓶子的倾角,瓶子倒空的耗时会缩短。这增加了所谓的气泡紧缩频率(bubble pinch off frequency),相对增量取决于流体的热物理性子。
“我们的实验表明存在一个临界倾角,在此之后,连续增大瓶子倾角也不会令瓶子排空韶光连续减少,”Rohilla 说,“这是由于空隙的饱和造成的,在瓶口处,液体周围的空气以倾斜的角度霸占了空间。”
研究确定了两种不同的倒空瓶模式。在一种模式下,由于瓶内气泡高频紧缩,液体排空速率增加。在另一种模式中,瓶内气泡体积增大,但产生频率相对较低,这也能使得液体排空频率增加。
Rohilla 说:“我们还创造,当一个垂直颠倒的瓶子排出液体时,会产生密封的气泡。与直觉相反,密封气泡与液体分离的点产生在瓶口之外。此外,在无粘性流体中由于险些没有内摩擦,液体层变薄,故而存在强烈喷射;而在粘性流体中完备不存在这种喷溅,气泡存在可控的周期性。”
密封气泡的形成。图片来源:Lokesh Rohilla
这项事情证明了瓶子的几何形状和热物理性子在缩短瓶子排空韶光方面发挥着浸染。
Das 说:“我们可以通过调度瓶子的几何形状来掌握瓶子的排放模式。直不雅观的、针对特定产品设计的的瓶子将能更好地掌握其排空速率。”
这种对瓶子几何形状更深刻的理解,将有助于饮料工业和化工厂等实际运用。
来源链接:
https://eurekalert.org/pub_releases/2020-04/aiop-bdr040620.php
论文信息
【标题】Fluidics in an emptying bottle during breaking and making of interacting interfaces
【作者】Lokesh Rohilla and Arup Kumar Dasa
【韶光】07 April 2020
【期刊】Physics of Fluids
【DOI】https://doi.org/10.1063/5.0002249
【择要】An experimental effort has been reported in the present study analyzing the fluidics during the emptying of a bottle. The viscosity of the containing liquid and the orientation of the bottle while emptying are varied to obtain different emptying modes. Stages during the emptying of a vertical upended and an inclined bottle have been demarcated based on prompt flow features. Fluidic phenomena such as formation and pinch-off of an encapsulated bubble, ejector jet, flooding, and stratification have been observed in a vertically upended bottle. The rise velocity, collapse dynamics, and growth rate of the bubble at the bottle mouth are affected by the angle of inclination and mainly viscosity of the emptying liquid. Two distinct bottle emptying modes have been identified in one of which the discharge rate is increased due to a high-frequency pinch-off of air bubbles inside the bottle and in another mode due to an increase in the volume of the pinched-off bubble at a comparatively lower frequency. The interaction of dominant forces during the emptying process has been established by quantifying Reynolds number, Weber number, and Bond number. For all emptying liquids, bottle emptying time reduces linearly up to a critical angle of inclination, θcrit ∼ 20°, and further follows an asymptote. We hypothesize that the transition between the linear regime and the asymptotic regime is due to the saturation of the voidage of the air at the cylindrical section of the bottle mouth. Furthermore, the geometry of the bottle also facilitates the growth rate of the bubble inside the bottle at θcrit.
本文来自微信"大众年夜众号“科研圈”,如需转载请联系 keyanquan@huanqiukexue.com
来源:科研圈
编辑:米老猫
↓ 点击标题即可查看 ↓
1. 为什么不倒翁小姐姐能摇一晚上不倒?
2. 如果在家考,作弊就很随意马虎吗?
3. 「我给你 37 美元,求求你把这桶油搬走吧」
4. 老师隔离后用射击游戏上网课,真不怕学生看完更想玩游戏?
5. 被五步蛇咬,走几步才最安全?
6. 我们从不骗你,除非……你不懂物理
7. 为什么菜油倒海上能救命?这道题美国学霸国父也不会做
8. 为什么有 32 个关卡的超级马里奥兄弟只要 64KB?
9. 十大物理效应,一次看个够!
10. 方程 E = mc² 中,m 的能量从何而来?